Analytic number theory
-
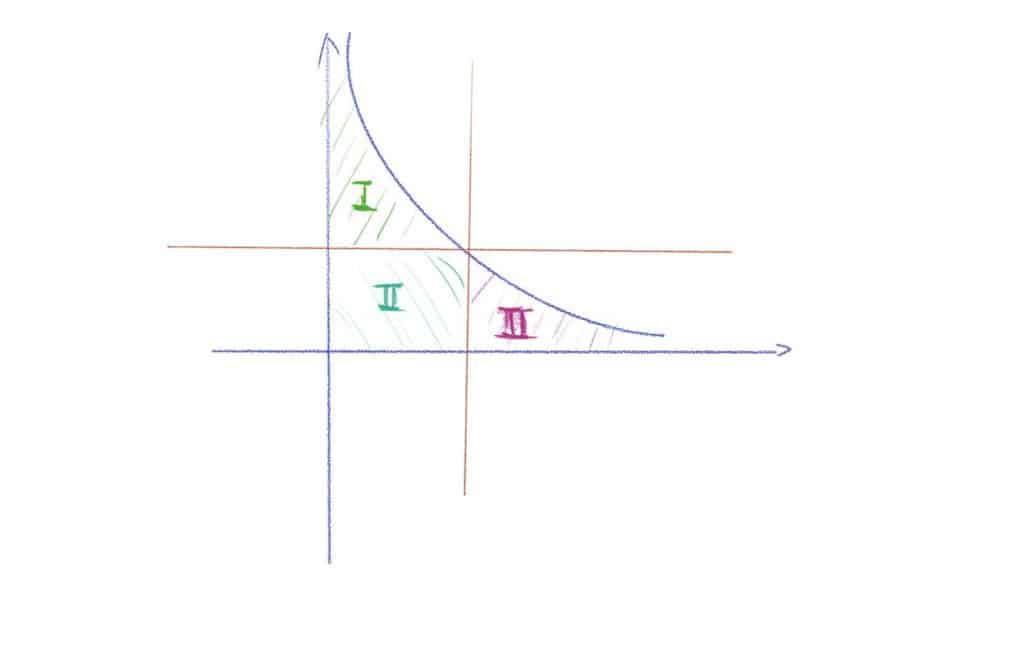
Dirichlet hyperbola method
A pdf version is Dirichlet hyperbola method. 1. Introduction Theorem 1 $\sum_{1\…
-
The large sieve and the Bombieri-Vinogradov theorem
-1.Motivation- Large sieve a philosophy reflect as a large group of inequalities…
-
The correlation of Mobius function and nil-sequences in short interval
I wish to establish the following estimate: Conjecture :(correlation of Mobius f…
-
Diophantine approximation of algebraic number
An important theorem in Diophantine approximation is the theorem of Liuoville: *…
-
Multiplication function on short interval
The most important beakgrouth of analytic number theory is the new understanding…
-
An approach to Vinogradov estimate
Vinogradov estimate is: $ |\sum_{n=1}^{N}e^{2\pi i\alpha P(n)}|\leq c_A\frac{N}{…
-
Covering a non-closed interval by disjoint closed intervals
this note will talk about the Ostrowski representation and approximation by cont…
-
Heat flow and the zero of polynomial-a approach to Riemann Hypesis
There is a main obstacle to improve the kakeya conjecture,remain in dimension 3,…
-
Bourgain-Sarnak-Ziegler Criterion
Bourgain-Sarnak-Ziegler定理可以视为Vingrodov均值定理的有限版本。
-
Sarnak猜想在skew product上的情形。
Cylinder map: Cylender map:这是一个动力系统$ \Theta=(T,T^2)$,$ T:T^2\longrightarrow T^2 …
